Summer flew by, as usual
Will I ever learn my lesson? Probably not. I suspect most people in math have the same problem: you buy a new book and have all sorts of grand plans about going through it and mastering all its theorems, etc. etc. That was me in February, when I ordered a copy of Differential Geometry of Curves and Surfaces by Kristopher Tapp.

Classical differential geometry has interested me since I first learned calculus and read a passage about curvature in an old text I used. When it came in the mail, I figured that would be my summer reading material; maybe not all of it, but a good portion of it. Well, you can make plans, but reality happens anyway.
I got through the "of curves" part, but "and surfaces" will mostly have to wait, it seems. This is a good book, and I would say it's accessible, but sometimes math is just hard and takes time to work through, especially when you are self-studying and have other things going on in life. Anyway, the material is just too deep to master on a first read-through. At the very least, going through the development of the Frenet frame is an experience that will stick with me.
What else have I been up to? Well, back in May I started with a fresh composition book for all of my excursions, and it's about halfway filled now with various scribblings. During the school year, there just isn't enough time to look at everything I'm interested in, so a lot of my reading this summer has focused on the stuff I have had to skip.
I've certainly left some clues that playing around with Maxima has kept me busy of late. I enjoy using computer algebra as a tool; I can work on the overall path toward a solution and leave the really hideous calculations to a computer. Maxima also has some good numerical facilities available, such as differential equation solvers, numerical root-finding capabilities, and polynomial interpolation. Differential equations can get hairy really quickly, even the ones used to model relatively simply situations in physics.
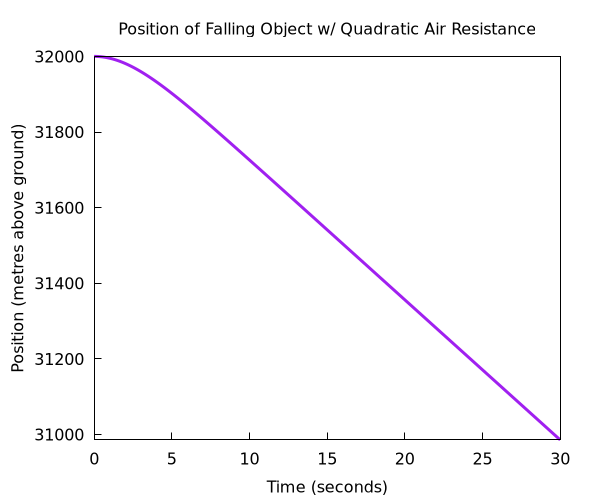
For example, one problem I enjoyed spending time on came from a mechanics text I have1, and really calls for a computer as you progress through increasingly complicated models of an object falling through the atmosphere. You can assume no air resistance and constant gravity, and the equation is easy to solve by hand (you can go straight to a kinematics equation to find the time of fall). Make it a little more complicated with some quadratic air resistance, \(\frac{1}{2}v|v|\), and that leads to a nonlinear differential equation that could still be solved by hand, with a tanh substitution. This is sort of at the limit of what can realistically be solved analytically by a student.
Then the fun begins. Introduce a much more complex model, where the air resistance depends on the density of the atmosphere at the particle's current position, and gravity depends on distance from the centre of the Earth. The resulting equation of motion is quite something:
\[\ddot{y} = -\frac{9.8}{\left(1+\frac{y}{6370000}\right)^2} + \frac{1}{2m}\exp\left(-\frac{y}{8000}\right)v^2.\]
I saw a few possibilities for massaging this into something tractable, but I wouldn't recommend spending too much time on it. As far as I can tell, there is an analytic2 solution which involves the exponential integral/incomplete gamma function.
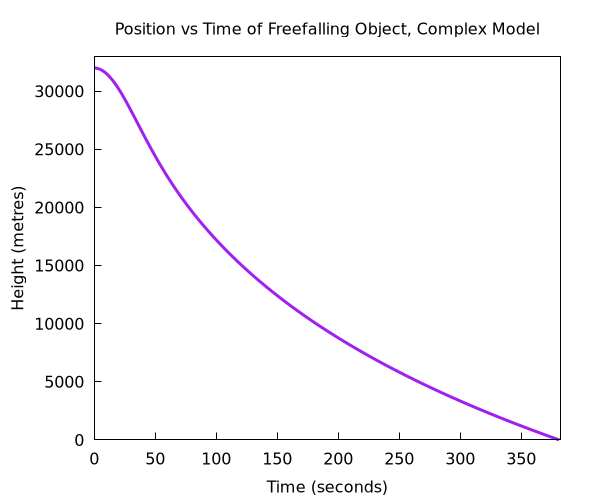
The above plot is from the numerical solution obtained with Maxima. It is an interesting curve, and that definitely seems like some kind of special function. This is not your grandfather's tanh function, I can tell you that much. The plot goes on for quite a while because the object takes a long time to fall under this model. This was sort of a new problem for me—with no explicit formula and a hard time eyeballing the plot, how would one estimate the root of this function? Maxima could generate an interpolating polynomial with the interpol package, and then find the root of that using Newton's method. It takes about 381 seconds, or 6.35 minutes.
I thought it was an interesting problem, and possibly inspired by the real-life Project Excelsior, where Joseph Kittinger jumped from a height of 31,333 metres. He took 13 minutes and 45 seconds to return to the ground level, but of course Kittinger used a parachute. (Something tells me parachute drag is a lot more complicated to model than what they teach in undergrad mechanics.)
Bookworm business aside, I always try to make the most of summer. See, here I am with my new car! (Not really, this is at the Ashton Brewpub in May where I had some delicious cider.).

Nothing like a bike ride in the summer along the Ottawa River. Some of the famous Remic Rapids rock sculptures…

My husband and I also celebrated our anniversary! We spend a wonderful sunny weekend on stay-cation at the hotel where our original reception was held, making new memories together.

Lots happened for me this summer, but there is still a bit more left. For the next two weeks I'm participating in the uOttawa Summer School, a multidisciplinary get-together of undergrads and early master students in mathematics and the sciences from around Canada (and maybe other places?)